M Jak Milosc Nowe Odcinki

round prisms and pyramids

revolution Solid

Round body limited by a generator (g) curve, which rotates around an axis (e). These may include:

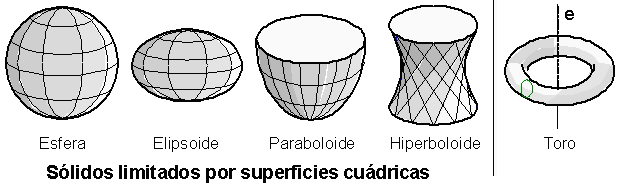
• solid surfaces limitdos by quadrics :
  • area: the generating station is a circle,
  • ellipsoid: the generating station is an ellipse,
  • paraboloid: the generating station is a parable,
  • hyperboloid: the generating station is a hyperbola,
• torus (ring) . Its surface is generated by a circle or an ellipse, which revolves around an axis (e), coplanar with it, and outside it.

Cylinder

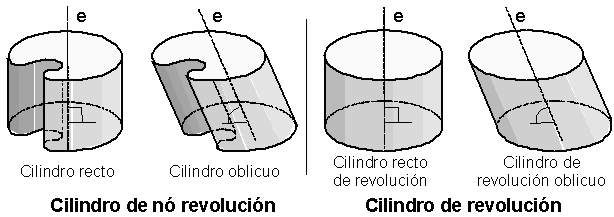
Round body limited by a cylindrical surface and two parallel flat bases. The line through the geometric centers of the bases is called the cylinder axis (e), and is parallel to the generatrix (g) of the cylindrical surface. Cylinders can be:

• right cylinder: if the axis (e) is perpendicular to the bases,
• Cilinder oblique ro: if the axis (e) is not perpendicular to the bases,
• c ilindro revolution: If you are limited by a cylindrical surface of revolution. Can in turn be:
  
  
  • straight cylinder revolution: if the axis (e) is perpendicular to the bases,
  • oblique cylinder revolution : if the axis (e) is not perpendicular to the bases.
• 
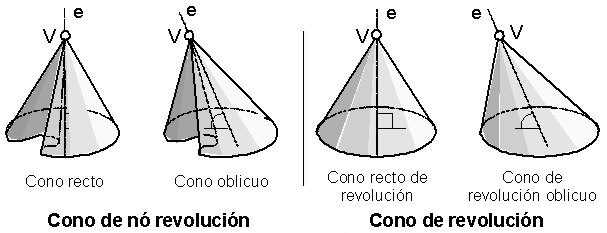
  Cone Round body limited by a conical surface and a flat base. The line through the vertex (V) of the conical surface and the geometric center of the base is called the cone axis (e). The cones can be:
• right cone: if the axis (e) is perpendicular to the base,
• oblique cone: if the axis (e) is not perpendicular to the base,
• cone of revolution : If you are limited by a conical surface of revolution. Can in turn be:
  • right cone of revolution : if the axis (e) is perpendicular to the base,
  • oblique cone of revolution : if the axis (e ) is not perpendicular to the base.